Classifications
conic
conformal
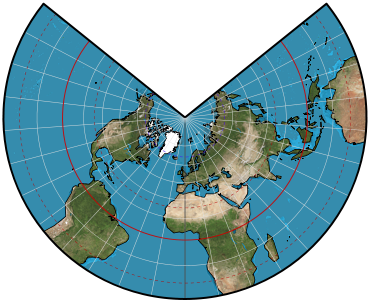
Graticule
Meridians: Equally spaced straight lines converging at a common point, which is one of the poles. The angles between them are less than the true angles.
Parallels: Unequally spaced concentric circular arcs centered on the pole of convergence of the meridians, which are therefore radii of the circular arcs. Spacing of parallels increases away from the central latitudes.
Poles: The pole nearest a standard parallel is a point; the other pole cannot be shown.
Symmetry: About any meridian.
Aspects
Bipolar oblique conic conformal projection is the only common oblique aspect.
Limiting forms
Polar Stereographic projection, if a pole is the single standard parallel. The cone of projection thereby becomes a plane.
Mercator projection, if the single standard parallel is the equator or if two standard parallels are symmetrically placed north and south of the equator. The cone of projection thereby becomes a cylinder. Standard conic formulas must be rewritten.
Scale
True along one or two chosen standard parallels, usually but not necessarily on the same side of the equator. As a rule of thumb, these parallels can be placed at one-sixth and five-sixths of the range of latitudes, but there are more refined means of selection. Scale is constant along any given parallel and is the same in all directions at a given point.
Distortion
Free of distortion only along the one or two standard parallels. Distortion is constant along any given parallel. Conformal everywhere except at the poles.
Usage
Extensively used in ellipsoidal form for large scale mapping of regions of predominantly east-west extent, including topographic quadrangles (1:24,000 and 1:62,500 scale) for many of the U.S. State Plane Coordinate System zones, many maps in the International Map of the World (1:1,000,000 scale) series, the U.S. State Base Maps (1:500,000 scale), and topographic mapping in many other nations.
Also used for atlas maps of some countries.
Recommended for conformal mapping of regions of predominantly east-west extent.
Similar projections
Various methods of determining optimum standard parallels have been proposed by John Herschel in 1860, V.V. Vitkovskiy (projection III) in 1907, N.Ya. Tsinger in 1916, and V.V. Kavrayskiy (projection III) in 1934. Once the standard parallels are selected, all these projections are constructed by using the same formulas used for the Lambert conformal conic with two standard parallels.
Bipolar oblique conic conformal projection consists of oblique aspects of two Lambert conformal conic projections laid side by side.
Origin
Presented by Johann Heinrich Lambert (1728–1777) of Alsace in 1772.
 Cylindric
Cylindric Pseudocylindric
Pseudocylindric Conic
Conic Azimuthal
Azimuthal Lenticular
Lenticular Miscellaneous
Miscellaneous